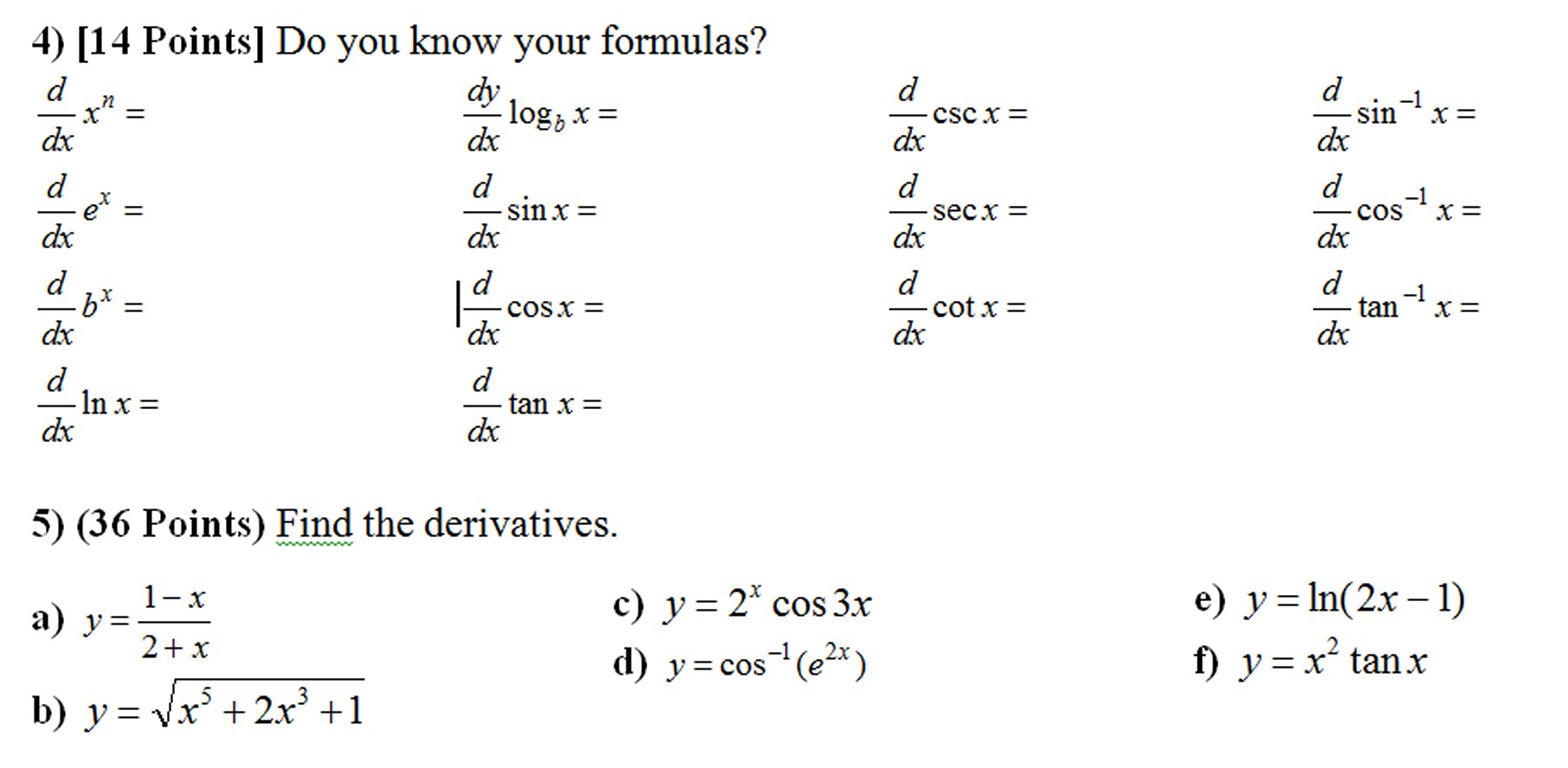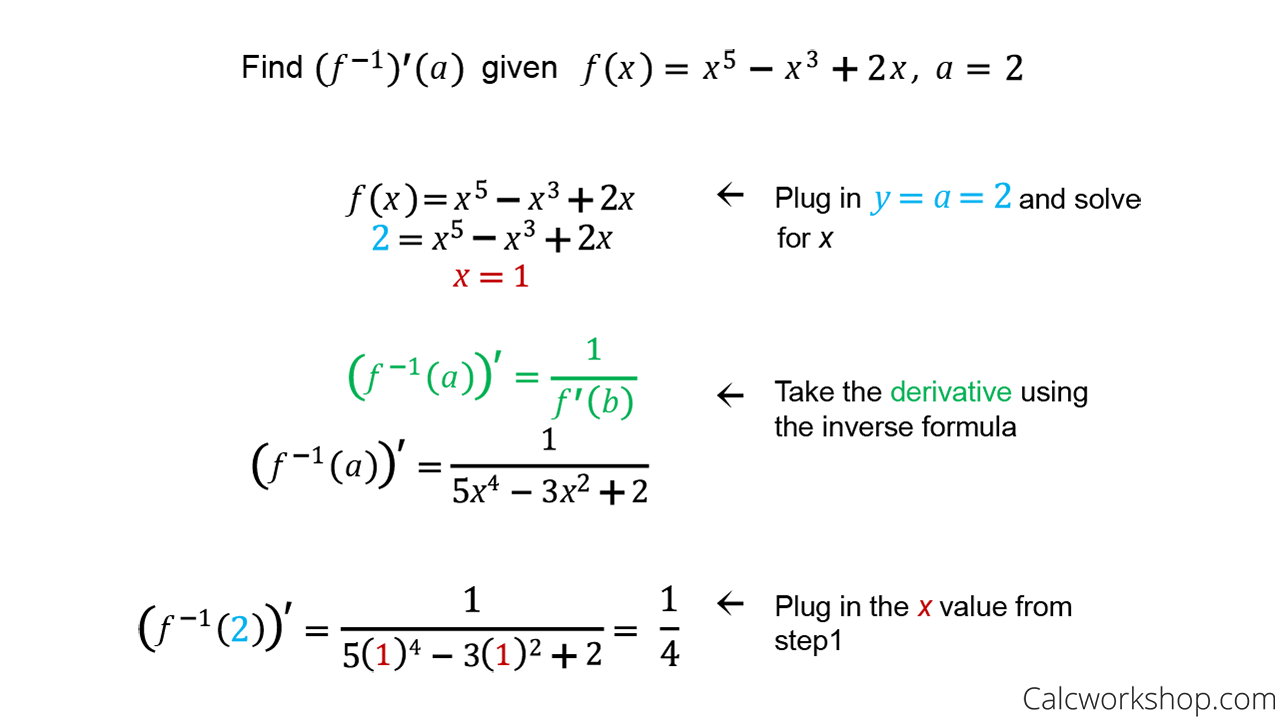The Secret Of Info About How To Get A Derivative

Δy δx = f (x+δx) − f (x) δx simplify it as best we can then make δx shrink towards zero. Find the derivatives of the following functions: Learn all about derivatives and how to find them here.

how to fix rod knock how to keep uterus healthy how to handle group conflict how to lose weight sitting down how to get out of boredom how to heal infected piercing how to get a ccw in florida how to gain a little weight

Question Video Finding The Derivative Of A Function Defined By An

Inverse Trig Derivatives (Derivatives of Inverse Trig Functions)

Inverse Trig Derivatives (derivatives Of Functions)

Graph The Derivative Youtube

How To Find The Derivative Of A Fraction Calculus Youtube

Finding Derivatives From A Graph Youtube

How to Calculate a Basic Derivative of a Function 9 Steps

How To Calculate A Basic Derivative Of Function 9 Steps

Yes, you said it!

How to get a derivative. Solution we use our new derivative rules to find. A classic example for second derivatives is found in basic physics. Calculus is the study of functions, and one useful attribute to know about a function is how fast it changes.

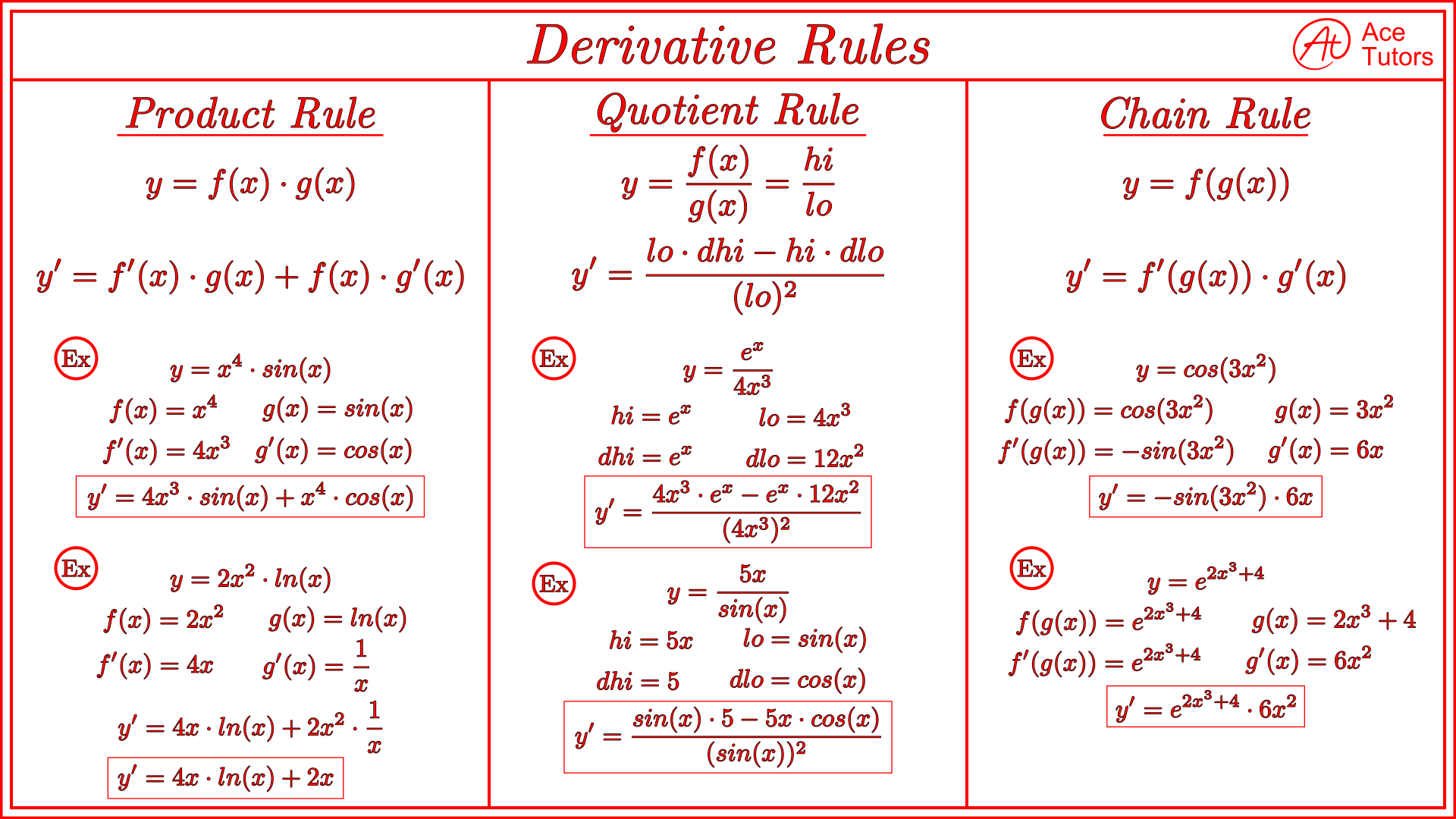
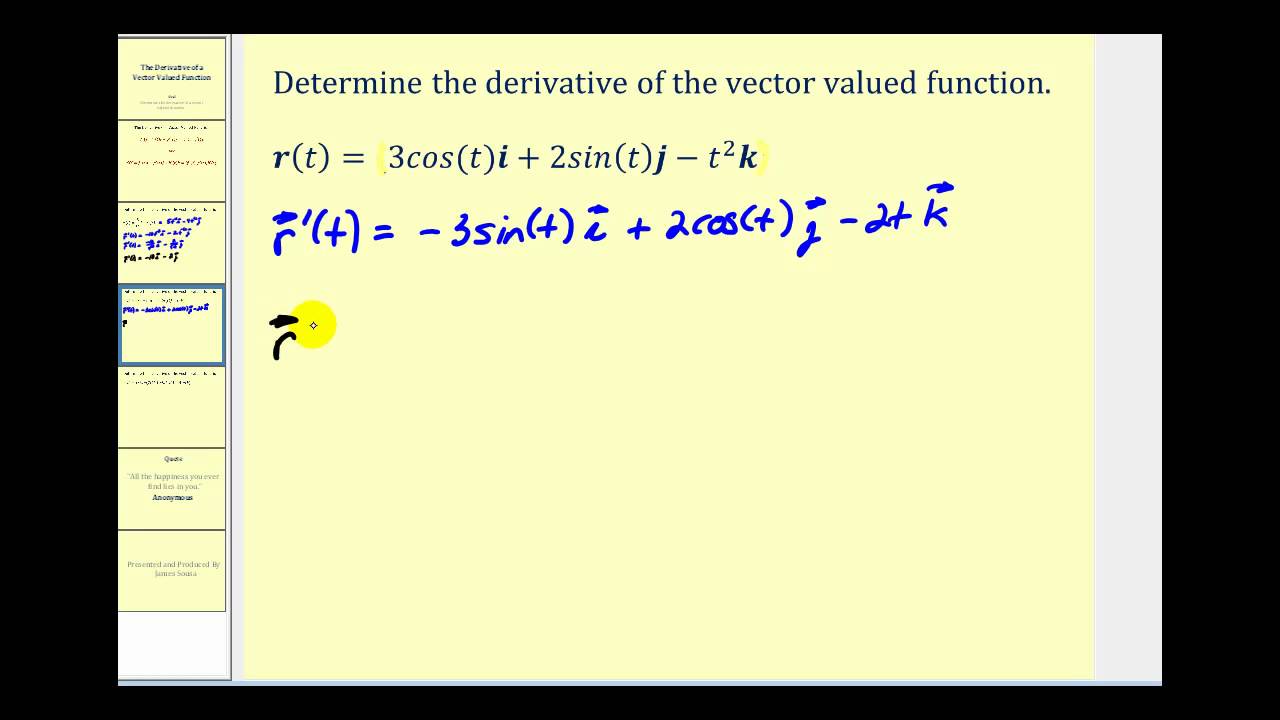
Multipliers and divisors), derive each component separately, carefully set the rule formula, and simplify. This year will be particularly busy for those focused on regulatory reporting. Derivative [ n1, n2,.] [ f] is the general form, representing a function obtained from f by differentiating n1 times with respect to the first argument, n2 times with respect to the second argument, and so on.

Represents the derivative of a function f of one argument. To solve this problem, we will use the following trigonometric identities and limits: Use f ′′(x) f ″ ( x) to find the second derivative and so on.

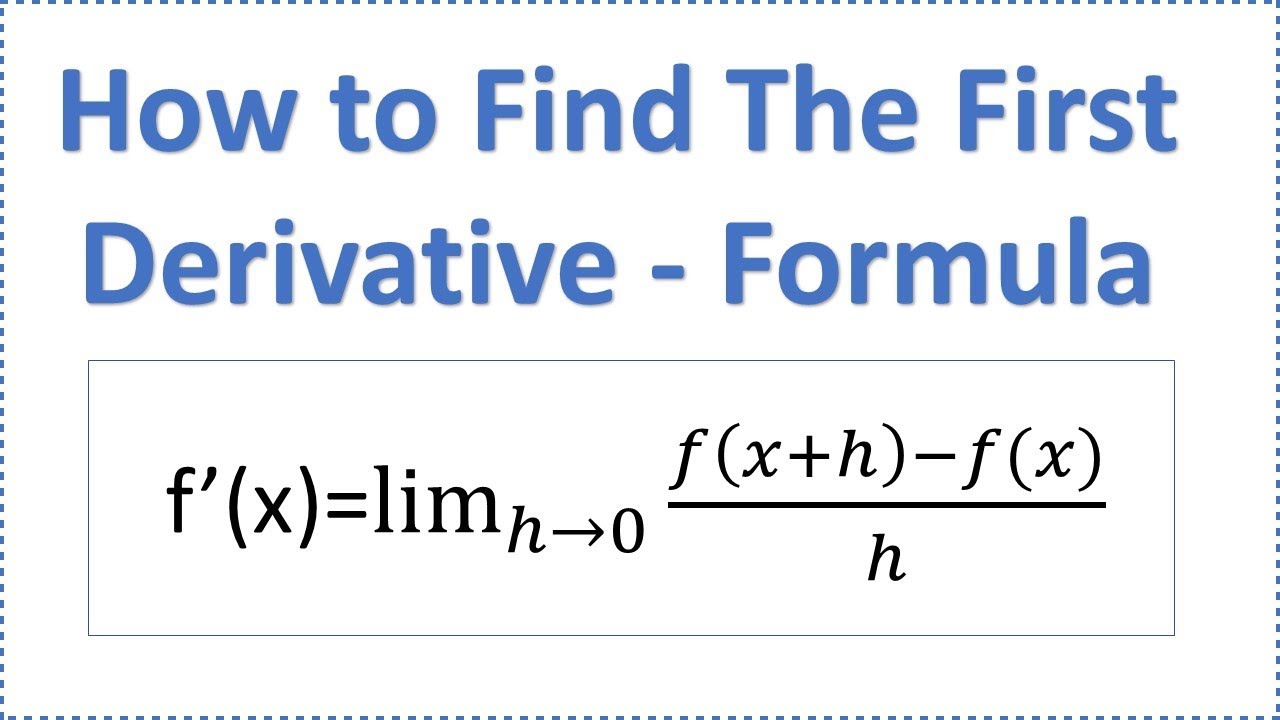
The derivative of a function describes the function's instantaneous rate of change at a certain point. Derivatives in calculus. Substitute your function into the limit definition formula.

To calculate derivatives start by identifying the different components (i.e. You can also get a better visual and understanding of the function by using our graphing tool. F' (x) = f' (1) = 2 (1) = 2 2.

Derivatives can be used to obtain useful characteristics about a function, such as its extrema and roots. Full 1 hour 35 minute video: Enter the function you want to find the derivative of in the editor.

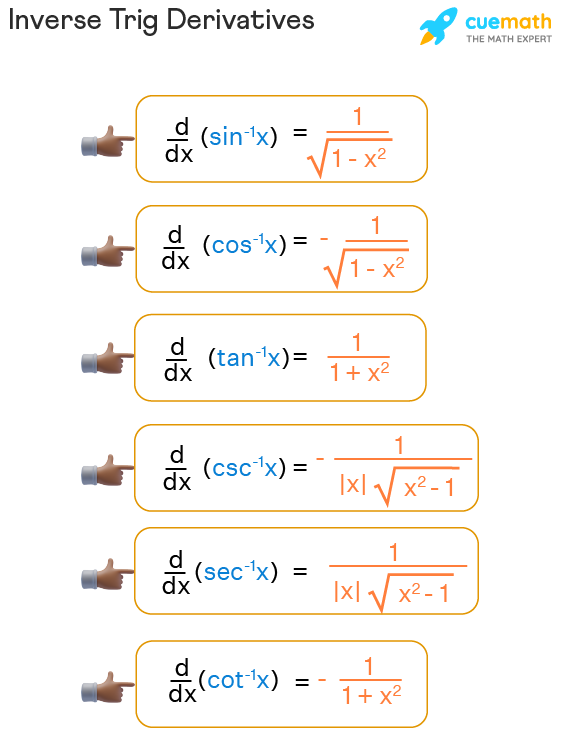
The important differentiation formulas are given below in the table. Example as a function, we take f (x) = x2. The first way of calculating the derivative of a function is by simply calculating the limit.

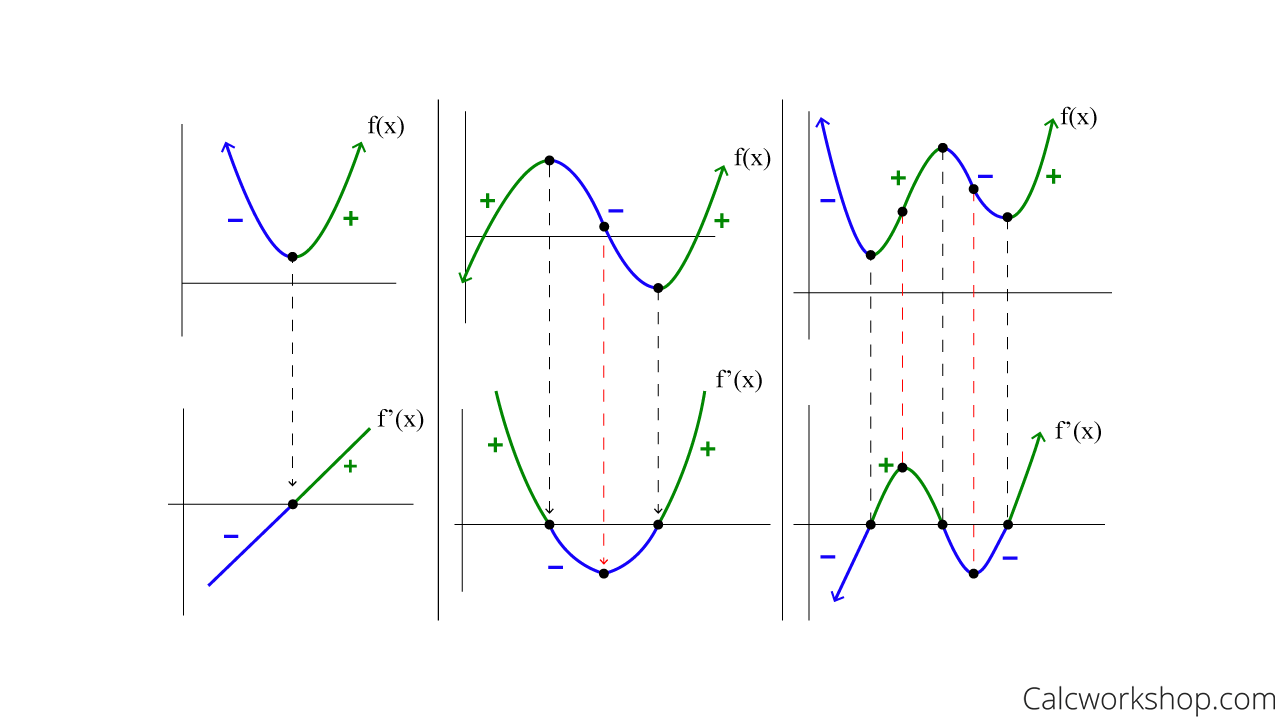
The derivative is an operator that finds the instantaneous rate of change of a quantity, usually a slope. Here, let us consider f (x) as a function and f' (x) is the derivative of the function. To find the derivative of a function y = f (x) we use the slope formula:

If f (x) = sin (x), then f' (x) = cos x. Let's explore how to find the derivative of any polynomial using the power rule and additional properties. To enter the prime symbol, you can click on the ' button located on standard keyboards.

The big idea of differential calculus is the concept of the derivative, which essentially gives us the direction, or rate of change, of a function at any of its points. Suppose we want to find the derivative of f. Another common interpretation is that the derivative gives us the slope of the line tangent to the function's graph at that point.