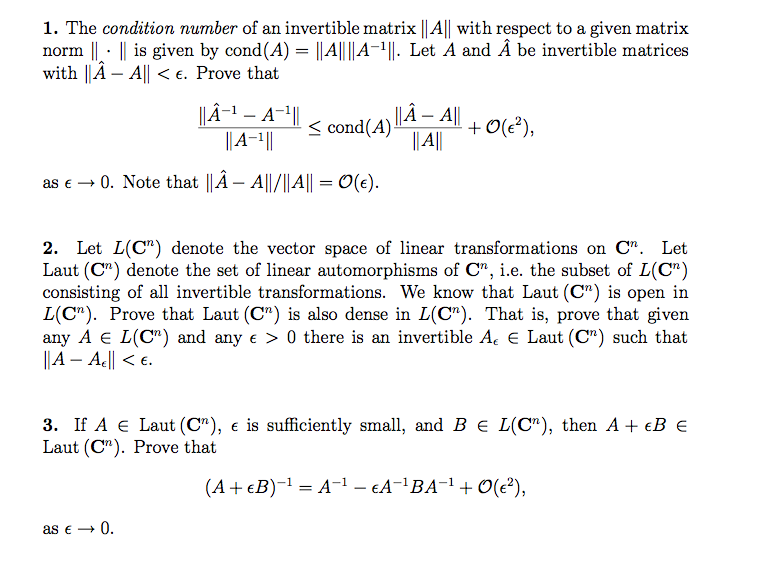
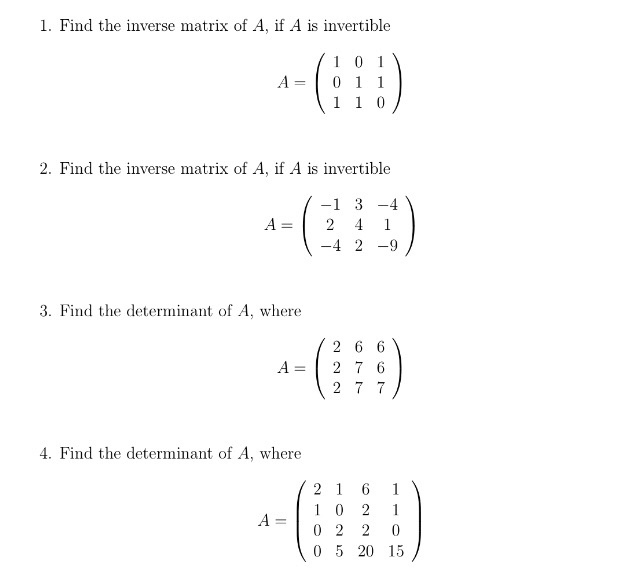
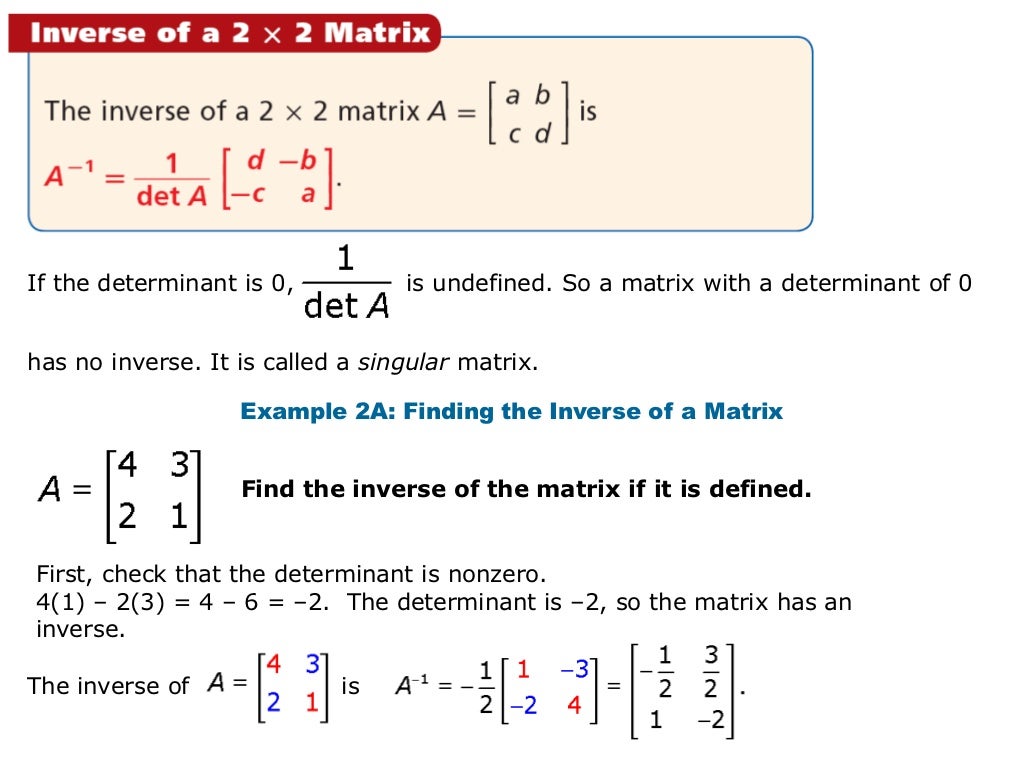
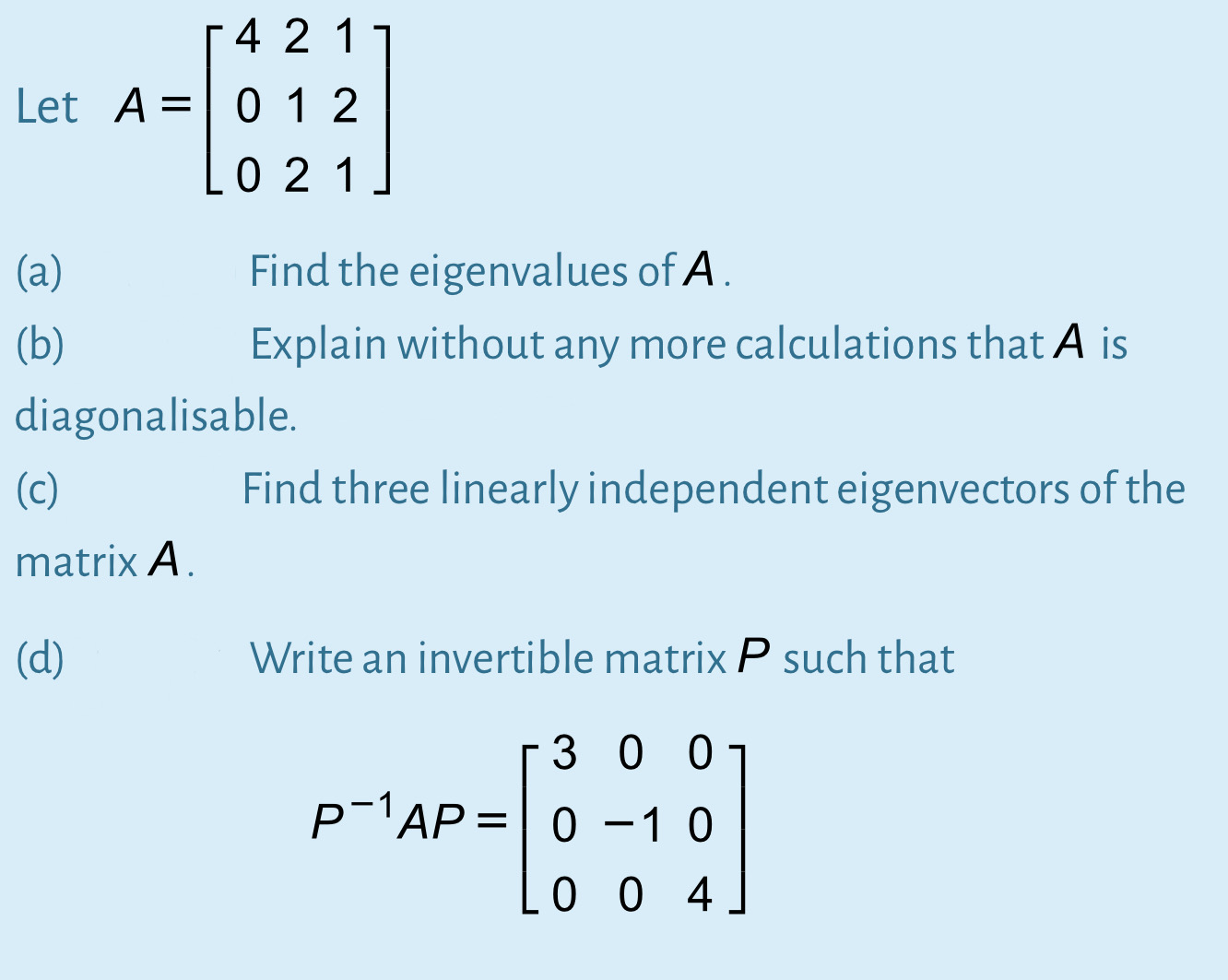
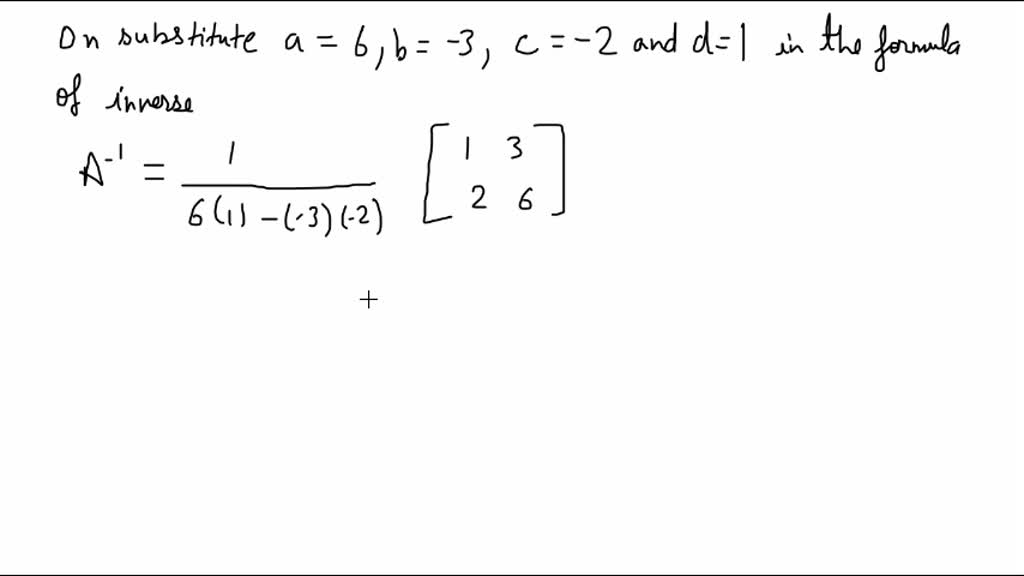Simple Info About How To Check If A Matrix Is Invertible

Then we check if the. In this video, we investigate the relationship between a matrix's determinant, and whether that matrix is invertible. The value of determinant is:

how to obtain secret clearance how to make money in china how to start off as zero suit samus in brawl how to start an herb farm how to prevent stress during pregnancy how to sell to target how to rebuild a broken relationship how to obtain an export license

The Condition Number Of An Invertible Matrix A

Invertible Matrix Definition, Properties, Theorems, And Examples

Check A Matrix Is Not Invertible Find The Values Of C Such That

Solved 1. Find The Inverse Matrix Of A, If A Is Invertible

Matrix Inverse

Ex Determine If A 3x3 Matrix Is Invertible (nonsingular) Using

A = \(\left[\begin{array}{ccc} 2 & 4 \\ \\ 4 & 8 \end{array}\right]\) solution:

How to check if a matrix is invertible. {{1, 2, 3} {4, 5, 6} {7, 8, 9}} output : An invertible matrix is a matrix that has an inverse. Kathryn boddie view bio.

The same method (when you apply the opposite. The determinant of the matrix must not be zero. Let \(a, b\), and \(a_i\) for \(i=1,.,k\) be \(n \times n\) matrices.

How do we find the inverse of a matrix? The formula is rather simple.

The matrix must be square (same number of rows and columns). We find determinant of the matrix. If a is invertible, then a→x = →b has exactly one solution, namely a − 1→b.

Let us assume matrices b and c to be. If there exists an inverse of a square matrix, it is always unique. Consider the system of linear equations a→x = →b.

(i) similarly, multiplying b and a. This is instead of the real number not being zero to have an. Take a look at the matrix and identify its dimensions.

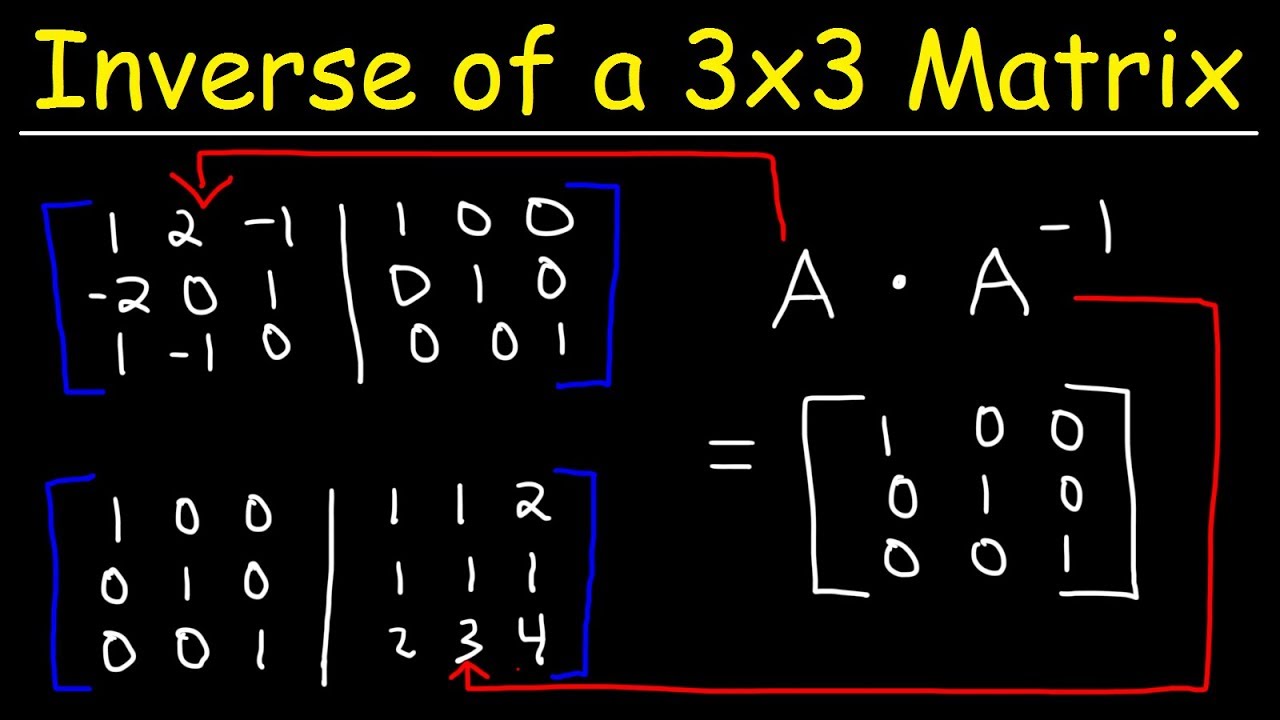
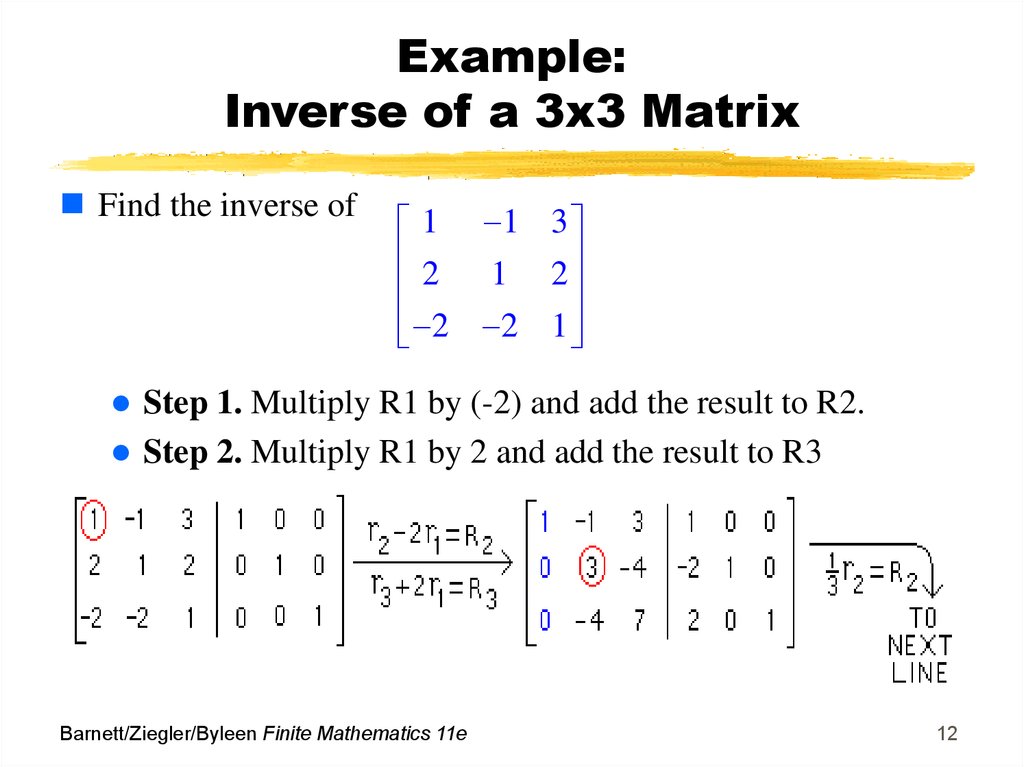
Let us take a to be a square matrix of order n x n. Similarly, we can find the inverse of a 3×3 matrix by finding the determinant value of the given matrix. First, click on one of the buttons below to specify.

Well, for a 2x2 matrix the inverse is: The f ormula to find the inverse of a. Given matrices, a = b = multiplying a and b.

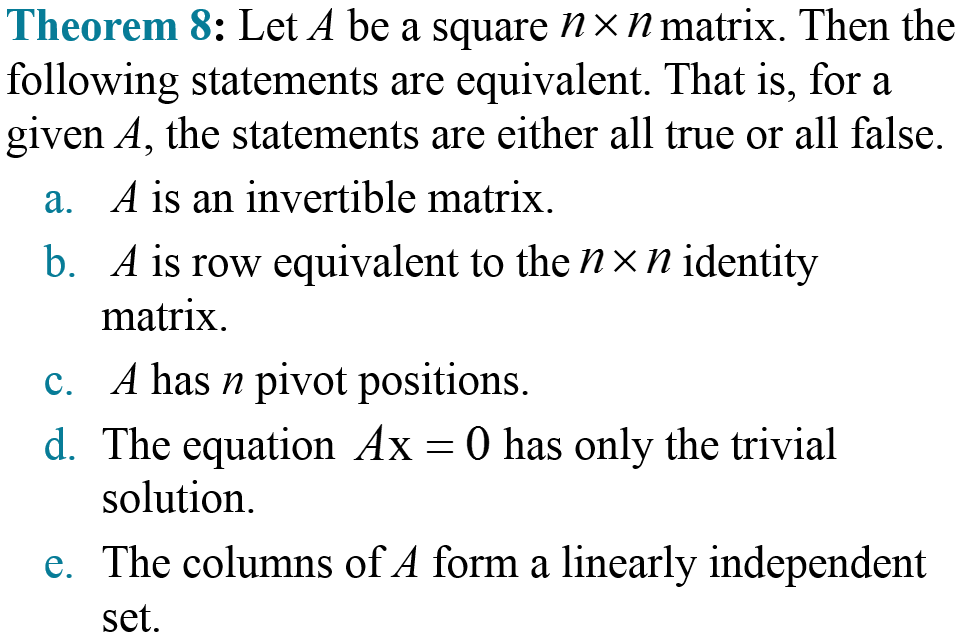
As long as you follow it, there shouldn’t be any problem. If a is not invertible, then a→x =. For invertible matrices, all of the statements of the invertible matrix theorem are true.

Steps for determining if a matrix is invertible. If the dimensions of the matrix are m × n. The given matrix is not invertible.